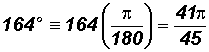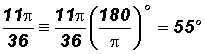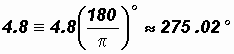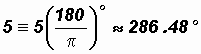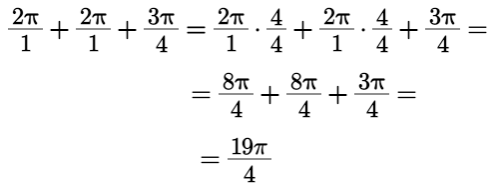INTRODUCTION TO ANGLES

Example 1:

If possible, express 164^o in EXACT radians ( is included in the answer!) reduced to lowest terms.

Given , we find the following:

 

Example 2:

Express 46.52^o in radians rounded to two decimal places. Use the button on your calculator instead of 3.14.

Given , we find the following:

Calculator Input: 46.52 / 180     Use the button on the calculator!

We find that 0.81 is its decimal approximation.

Example 3:

If possible, express the radian measure in EXACT degree measure.   Reduce to lowest terms if necessary.

Given , we find the following:

Example 4:

Express the radian measure in degree measure rounded to two decimal places. Use the button on your calculator instead of 3.14.

Given , we find the following:

NOTE:  Whenever possible, use the button on your calculator instead of 3.14 because this will result in more exact calculations.

Example 5:

Express the radian measure 4.8 in degree measure rounded to two decimal places. Use the button on your calculator instead of 3.14 .

Given , we find the following:

Example 6:

Express the radian measure 5 in degree measure rounded to two decimal places. Use the button on your calculator instead of 3.14 .

Given , we find the following:

Example 7:

If possible, express 630^o in EXACT radians ( is included in the answer!) reduced to lowest terms.

Given , we find the following:

Here we had to reduce fractions!

Alternative method since we notice that 630^o is a multiple of the quadrantal angle. It does not require reducing fractions.

We notice that 630^o = 7(90^o).

We know that . Therefore, we can immediately state that 7(90^o) is equivalent to or .

Example 8:

If possible, express 4 in EXACT degree measure.

Given , we find the following:

Here we had to reduce fractions!

Alternative method since we notice that 4 is a multiple of the quadrantal angle. It does not require reducing fractions.

1. We notice that 4is actually 4

We know that . Therefore, we can immediately state that 4is equivalent to 4(180^o) = 720^o.

2. We could also notice that 4= 2(2)

We know that . Therefore, we can immediately state that 4is equivalent to 2(360^o) = 720^o.

Example 9:

If possible, express 315^o in EXACT radians ( is included in the answer!) reduced to lowest terms.

Given , we find the following:

Here we had to reduce fractions!

Since we were asked to find EXACT radians we are going to leave the answer in terms of a fraction containing the number . 

Alternative method since we notice that 315^o is a multiple of a special angle. It does not require reducing fractions.

We notice that 315^o = 7(45^o).

We know that . Therefore, we can immediately state that 7(45^o) is equivalent to or .

Example 10:

If possible, express 330^o in EXACT radians ( is included in the answer!) reduced to lowest terms.

Given , we find the following:

Here we had to reduce fractions!

Alternative method since we notice that 330^o is a multiple of a special angle. It does not require reducing fractions.

We notice that 330^o = 11(30^o)

We know that . Therefore, we can immediately state that 11(30^o) is equivalent to or .

Example 11:

If possible, express 120^o in EXACT radians ( is included in the answer!) reduced to lowest terms.

Given , we find the following:

Here we had to reduce fractions!

Alternative method since we notice that 120^o is a multiple of a special angle. It does not require reducing fractions.

We notice that 120^o = 2(60^o)

We know that . Therefore, we can immediately state that 2(60^o) is equivalent to or .

Example 12:

If possible, express the radian measure in EXACT degree measure reduced to lowest terms.

Given , we find the following:

Here we had to reduce fractions!

Alternative method since we notice that is a multiple of a special angle. It does not require reducing fractions.

We notice that .

We know that . Therefore, we can immediately state that is equivalent to

4(60^o) = 240^o.

Example 13:

If possible, express the radian measure in EXACT degree measure reduced to lowest terms.

Given , we find the following:

Alternative method since we notice that t is a multiple of a special angle. It does not require reducing fractions.

We notice that .

We know that . Therefore, we can immediately state that is equivalent to

3(45^o) = 135^o.

Example 14:

Which angles are quadrantal angles?

All quadrantal angles are integer multiples of 90^o!

a. 388^o

4(90^o) + 28^o , QI angle

b. 450^o

5(90^o), quadrantal angle

c. 720^o

8(90^o), quadrantal angle

d. 390^o

4(90^o) + 30^o, QIII angle

e. 540^o

6(90^o), quadrantal angle

f. 225^o

2(90^o) 45^o, QII angle

Example 15:

Which angles are quadrantal angles? Sometimes it is easier to change the angles to degrees before you decide!

All quadrantal angles are integer multiples of /2 90^o!

a. /8

22.5^o, QI angle

b. 5/2

5/2 590^o, quadrantal angle

c. 3/2

3/2 390^o, quadrantal angle

d. 6/5

216^o, QIII angle

e.

2/2 290^o, quadrantal angle

Example 16:

Which angles are integer multiples of special angles?

Special angles are 30^o, 45^o, and 60^o.

a. 388^o

not a multiple of special angle since neither 30, nor 45, nor 60 divides into angle evenly

b. 330^o

11(30^o), multiple of special angle

c. 225^o

5(45^o), multiple of special angle

d. 119^o

not a multiple of special angle since neither 30, nor 45, nor 60 divides into angle evenly

e. 120^o

2(60^o), multiple of special angle

f. 150^o

5(30^o), multiple of special angle

Example 17:

Which angles are integer multiples of special angles? Sometimes it is easier to change the angles to degrees before you decide!

Special angles are 30^o /6 and 45^o /4 and 60^o /3.

a. /8

22.5^o, not a special angle

b. 5/3

5/3 560^o, multiple of special angle

c. 3/4

3/4 345^o, multiple of special angle

d. /5

36^o, not a special angle

e. 7/6

7/6 730^o, multiple of special angle

f. 4/9

80^o, not a special angle

Example 18:

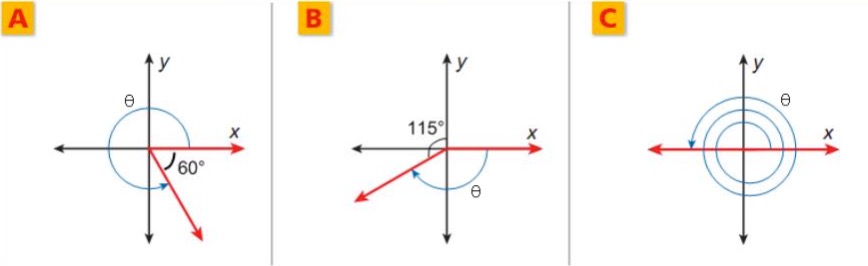
Find the angle measures from each graph.

A.

= 360^o 60^o = 300^o

B.

Here we must first find the angle between the terminal side of angle and the negative x-axis. Given is 115^o. We can change it to the sum of 90^o and 25^o. Therefore, tha angle between the terminal side of and the negative x-axis is 25^o.

Then = 180^o +25^o = 155^o

C.

We see that angle has two full rotations, which equals 360^o(2). Remaining is a rotation of 180^o.

Then = 360^o(2) +180^o = 900^o

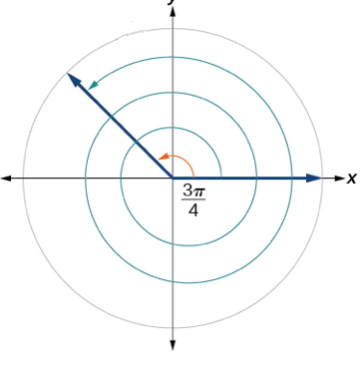
Example 19:

Find the measure of the angle below in radians. Express the angle as an improper fraction in terms of .

The direction of the angle is counter-clockwise. Therefore, we are dealing with a positive angle. We are counting two full rotations which is 2 + 2 = 4. To it we will add the measure of the arc .

We are supposed to express the answer as an improper fraction in terms of . When adding fractions, we need a common denominator. In our case, it is 4.

The measure of the angle in radians is .

Example 20:

Find the location of the terminal side of the following angles.

Hint: Graph them!

*********************************************************************************************

a.      77o - Quadrant I angle	b.     77o - Quadrant IV angle
c.   125o  - Quadrant II angle	d.   125o - Quadrant III angle
e.   216o  - Quadrant III angle	f.    216o - Quadrant II angle
g.   330o - Quadrant IV angle i.   960o = 360o(2) + 240o     Quadrant III angle using the angle 240o     which is coterminal to the angle 960o	h.   330o - Quadrant I angle   j.   376o = 360o(1) + (16o)       Quadrant IV angle using the angle 16o       which is coterminal to the angle 376o

Example 21:

Find the location of the terminal side of the following angles.

Hint: If necessary, convert the angles to decimal form, then graph them.

Remember that   and    and    and  .

*********************************************************************************************